How Brakes Work

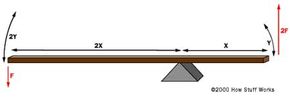
­In the fi­gure below, a force F is being applied to the left end of the lever. The left end of the lever is twice as long (2X) as the right end (X). Therefore, on the right end of the lever a force of 2F is available, but it acts through half of the distance (Y) that the left end moves (2Y). Changing the relative lengths of the left and right ends of the lever changes the multipliers.

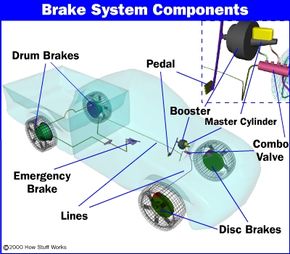
The basic idea behind any hydraulic system is very simple: Force applied at one point is transmitted to another point using an incompressible fluid, almost always an oil of some sort. Most brake systems also multiply the force in the process.

Simple hydraulic system

Two pistons are fit into two glass cylinders filled with oil and connected to one another with an oil-filled pipe. If you apply a downward force to one piston, then the force is transmitted to the second piston through the oil in the pipe. Since oil is incompressible, the efficiency is very good -- almost all of the applied force appears at the second piston. The great thing about hydraulic systems is that the pipe connecting the two cylinders can be any length and shape, allowing it to snake through all sorts of things separating the two pistons. The pipe can also fork, so that one master cylinder can drive more than one slave cylinder if desired.

Master cylinder with two slaves

The other neat thing about a hydraulic system is that it makes force multiplication (or division) fairly easy. If you have read How a Block and Tackle Works or How Gear Ratios Work, then you know that trading force for distance is very common in mechanical systems. In a hydraulic system, all you have to do is change the size of one piston and cylinder relative to the other.

Hydraulic multiplication

To determine the multiplication factor, start by looking at the size of the pistons. Assume that the piston on the left is 2 inches (5.08 cm) in diameter (1-inch / 2.54 cm radius), while the piston on the right is 6 inches (15.24 cm) in diameter (3-inch / 7.62 cm radius). The area of the two pistons is Pi * r². The area of the left piston is therefore 3.14, while the area of the piston on the right is 28.26. The piston on the right is nine times larger than the piston on the left. This means that any force applied to the left-hand piston will come out nine times greater on the right-hand piston. So, if you apply a 100-pound downward force to the left piston, a 900-pound upward force will appear on the right. The only catch is that you will have to depress the left piston 9 inches (22.86 cm) to raise the right piston 1 inch (2.54 cm).

Next, we'll look at the role that friction plays in brake systems.

­Friction is a measure of how hard it is to slide one object over another. Take a look at the figure below. Both of the blocks are made from the same material, but one is heavier. I think we all know which one will be harder for the bulldozer to push.

To understand why this is, let's take a close look at one of the blocks and the table:

Even though the blocks look smooth to the naked eye, they are actually quite rough at the microscopic level. When you set the block down on the table, the little peaks and valleys get squished together, and some of them may actually weld together. The weight of the heavier block causes it to squish together more, so it is even harder to slide.

Different materials have different microscopic structures; for instance, it is harder to slide rubber against rubber than it is to slide steel against steel. The type of material determines the coefficient of friction, the ratio of the force required to slide the block to the block's weight. If the coefficient were 1.0 in our example, then it would take 100 pounds of force to slide the 100-pound (45 kg) block, or 400 pounds (180 kg) of force to slide the 400-pound block. If the coefficient were 0.1, then it would take 10 pounds of force to slide to the 100-pound block or 40 pounds of force to slide the 400-pound block.

So the amount of force it takes to move a given block is proportional to that block's weight. The more weight, the more force required. This concept applies for devices like brakes and clutches, where a pad is pressed against a spinning disc. The more force that presses on the pad, the greater the stopping force.

Let's say for example that the distance from the pedal to the pivot is four times the distance from the cylinder to the pivot, so the force at the pedal will be increased by a factor of four before it is transmitted to the cylinder.

Also if the diameter of the brake cylinder is three times the diameter of the pedal cylinder. This further multiplies the force by nine. All together, this system increases the force of your foot by a factor of 36. If you put 10 pounds of force on the pedal, 360 pounds (162 kg) will be generated at the wheel squeezing the brake pads.

There are a couple of problems with this simple system. What if we have a leak? If it is a slow leak, eventually there will not be enough fluid left to fill the brake cylinder, and the brakes will not function. If it is a major leak, then the first time you apply the brakes all of the fluid will squirt out the leak and you will have complete brake failure.

The master cylinder on modern cars is designed to deal with these potential failures. Be sure to check out the article on How Master Cylinders and Combination Valves Work, and the rest of the articles in the brake series (see the links on the next page), to learn more.